Class 10 maths ex 7.1 solutions
Table of Contents
Co-Ordinate geometry class 10 exercise 7.1
Class 10 Maths Exercise 7.1 solutions : We are providing Class 10 Maths solutions for 7th chapter ex 7.1 class 10 maths “Co-ordinate Geometry” textbook provided by NCERT for CBSE, State boards etc complete exercise solutions with Additional Most Important Questions in our website – online, absolutely for FREE for everyone. No need to do any sign in process, just browse and enjoy all of the Class 10 Maths Chapter 7 exercise 7.1 solutions from us. From the First exercise “Real Numbers”, there are total 4 exercise given in bellow table. Click on the exercise number to browse the complete solutions from ex 7.1 class 10 or the Class 10 Maths Exercise 7.1 solutions ..
You are at co-ordinategeometry Exercise 7.1
Go to co-ordinate geometry Exercise 7.2
Go to co-ordinate geometry Exercise 7.3
Sub-Contents of “Chapter 7 Co-Ordinate Geometry” : There are total 6 sub contents exists in Class 10 maths chapter 7 Co-Ordinate Geometry . We need to study all of the sub-contents first. The Sub Contents are-
| 1. Introduction |
| 2. Distance Formula |
| 3. Section Formula |
| 4. Area of Triangle |
| 5. Summary |
| FROM LATEST UPDATED NCERT SYLLABUS |
Complete Class 10 Maths Exercise 7.1 solutions from latest updated NCERT Syllabus are given bellow:
Exercise 7.1 Class 10 MathsCo-Ordinate Geometry Class 10 Maths Ex 7.1 Question 1Find the distances between following pairs of points: (i) (2, 3), (4, 1) (ii) (-5, 7), (-1, 3) (iii) (a, b), (-a, -b) Solutions: (i) Distance between (2, 3) and (4, 1) is = \sqrt{(4 - 2)² + (1 - 3)²} = \sqrt{(2)² + (-2)²} = \sqrt{4 + 4} = √8 = 2√2 Therefore, Required Distance is 2√2 unites. (ii) Diastance between (-5. 7) and (-1, 3) is = \sqrt{(-1 - (-5))² + (3 - 7)²} = \sqrt{(-1 + 5)² + (-4)²} = \sqrt{(4)² + (-4)²} = \sqrt{16 + 16} = √32 = 4√2 Therefore, Required Distance = 4√2 unites. (iii) Distance between (a, b) and (-a, -b) is = \sqrt{ (-a - a)² + (-b - b)²} = \sqrt{ (-2a)² + (-2b)²} = \sqrt{ 4(a² + b²)} = 2 \sqrt{a² + b²} Therefore, The required distance is 2 \sqrt{a² + b²} unites.
Class 10 Maths Ex 7.1 Question 2Find the distance between (0, 0) and (36, 15). Solution: The distance between the origin and (36, 15) is = \sqrt{36² + 15²} = \sqrt{1296 + 225} = \sqrt{1521} = 39 So, Required distance between the given points is 39 unites. Class 10 Maths Ex 7.1 Question 3Determine the points (1, 5), (2, 3) and (-2, -11) are collinear. Solution: First Method: Let the points be A(1, 5), B(2, 3) and C(-2, -11) Now, Distance AB = \sqrt{(2 - 1)² + (3 - 5)²} = \sqrt{(1)² + (-2)²} = \sqrt{5} Distance BC = \sqrt{(-2 - 2)² + (-11 - 3)²} = \sqrt{(-4)² + (-14)²} = \sqrt{16 + 196} = \sqrt{212} = 2√53 Distance AC = \sqrt{(-2 - 1 )² + (-11 - 5 )²} = \sqrt{(-3)² + (-16)²} = \sqrt{9 + 256} = \sqrt{265} Clearly, AB + BC ≠ AC here. So, The given three are not collinear. 2nd Method: Here, x1 = 1, y1 = 5, x2 = 2, y2 = 3, x3 = -2, y3 = -11 Now, x1 (y2 – y3) + x2 (y3 – y1) + x3 (y1 – y2) = 1{3 – (-11)} + 2(-11 – 5) + (-2)(5 – 3) = 1 (3 + 11) + 2 × (-16) -2 × 2 = 14-32-4 = -22 ≠ 0 Therefore, The given three points will create a triangle, means the points are not collinear. Class 10 Maths Ex 7.1 Question 4Check whether the points (5, -2), (6, 4) and (7, -2) are the vertices of an isosceles triangle. Solution: Let the points be A(5, -2), B(6, 4) and C(7, -2) Distance AB = \sqrt{(6 - 5)² + (4 - (-2) )²} = \sqrt{(1)² + (6)²} = \sqrt{1 + 36} = \sqrt{37} Distance BC = \sqrt{(7 - 6)² + (-2 - 4 )²} = \sqrt{(1)² + (-6)²} = \sqrt{1 + 36} = \sqrt{37} We get, AB = BC So, The given three points are the vertices of a isosceles triangle.
Class 10 Maths Ex 7.1 Question 6Name the type of quadrilateral formed if any, by the following points, and give reasons for your answers.
Solutions: (i) let the points be P(-1, -2), Q(1, 0), R(-1, 2) and S(-3, 0) Distance PQ = \sqrt{(1 - (-1))² + (0 - (-2) )²} = \sqrt{(1 + 1)² + (0 + 2)²} = \sqrt{4 + 4} = \sqrt{8} = 2 \sqrt{2} Distance QR = \sqrt{(-1 - 1)² + (2 - 0)²} = \sqrt{(-2)² + (2)²} = \sqrt{4 + 4} = 2 \sqrt{2} Distance RS = \sqrt{(-3 - (-1))² + (0 - 2)²} = \sqrt{(-3 + 1)² + (-2)²} = \sqrt{4 + 4} = 2 \sqrt{2} Distance PS = \sqrt{(-3 - (-1))² + (0 - (-2))²} = \sqrt{(-3 + 1)² + (0 + 2)²} = \sqrt{4 + 4} = 2 \sqrt{2} So, we found that the lenghts of all the sides are equal. Diagonal PR = \sqrt{(-1 - (-1))² + (2 - (-2))²} = \sqrt{(-1 + 1)² + (2 + 2)²} = \sqrt{0 + 16} = 4 Diagonal QS = \sqrt{(-3 -1)² + (0 - 0)²} = \sqrt{16 + 0} = 4 Finally, we also found that the lenghts of diagonal are also same. This is the property of rhombus. So, The four given points will form a rhombus. (ii) Let the points be P(-3, 5), Q(3, 1), R(0, 3) and S(-1, -4) Distance PQ = \sqrt{(3 - (-3))² + (1 - 5)²} = \sqrt{(3 + 3)² + (-4)²} = \sqrt{36 + 16} = \sqrt{52} = 2 \sqrt{13} Distance QR = \sqrt{(0 - 3)² + (3 - 1)²} = \sqrt{(-3)² + (2)²} = \sqrt{9 + 4} = \sqrt{13} Distance RS = \sqrt{(-1 - 0)² + (-4 -3)²} = \sqrt{(-1)² + (-7)²} = \sqrt{1 + 49} = \sqrt{50} = 5 \sqrt{2} Distance PS = \sqrt{(-1 - (-3))² + (-4 -5)²} = \sqrt{(-1 + 3)² + (-9)²} = \sqrt{4 + 81} = \sqrt{85} Here, All the lenghts are unequal. So, The given points will not create any quadrilateral. (iii) Let the points be P(4, 5), Q(7, 6), R(4, 3) and S(1, 2) Distance PQ = \sqrt{(7 - 4)² + (6 - 5)²} = \sqrt{(3)² + (1)²} = \sqrt{9 + 1} = \sqrt{10} Distance QR = \sqrt{(4 - 7)² + (3 - 6)²} = \sqrt{(-3)² + (-3)²} = \sqrt{9 + 9} = 3 \sqrt{2} Distance RS = \sqrt{(1 - 4)² + (2 - 3)²} = \sqrt{(-3)² + (-1)²} = \sqrt{9 + 1} = \sqrt{10} Distance PS = \sqrt{(1 - 4)² + (2 -5)²} = \sqrt{(-3)² + (-3)²} = \sqrt{9 + 9} = 3 \sqrt{2} We see that the oposite sides are equal. Lets find the diagonal now, Diagonal PR = \sqrt{(4 - 4)² + (3 -5)²} = \sqrt{0 + 4} = 2 Diagonal QS = \sqrt{(1 - 7)² + (2 - 6)²} = \sqrt{(-6)² + (-4)²} = \sqrt{36 + 16} = \sqrt{52} We see that the diagonals are not equal. So, clearly, the formed quadrilateral is a Parallelogram.
Class 10 Maths Ex 7.1 Question 7Find the point on the x axis which is equidistant from (2, -5) and (-2, 9). Solution: Let the point on the X axis be (x, 0) Given, distance between (2, -5), (x, 0) = distance between (-2, 9), (x, 0) ⇒ \sqrt{(x - 2)²+(0 - (-5))²} = \sqrt{(x - (-2) )²+(0 - 9)²} ⇒ (x – 2)² + 5² = (x + 2)² + 9² [squaring both sides] ⇒ x² – 4x + 4 + 25 = x² + 4x + 4 + 81 ⇒ -4x -4x = 85 – 29 ⇒ -8x = 56 ⇒ x = \cfrac {56} {-8} ⇒ x = -7 Therefore, The required point on the x axis is (-7, 0). Class 10 Maths Ex 7.1 Question 8Find the value of y for which the distance between the points P(2, -3) and Q(10, y) is 10 unites. Solution: Given that, Distance Between P(2, -3) and Q(10, y) = 10 unites ⇒ \sqrt{(10 - 2)² + (y - (-3) )²} = 10 ⇒ (8)² + (y + 3)² = 100 [squaring both sides] ⇒ 64 + y² + 6y + 9 = 100 ⇒ y² + 6y + 73 – 100 = 0 ⇒ y² + 6y – 27 = 0 ⇒ y² +9y – 3y – 27 = 0 ⇒ y(y + 9) – 3(y + 9) = 0 ⇒ (y + 9)(y – 3) = 0 So, y + 9 = 0 OR y – 3 = 0 ⇒ y = – 9 OR y = 3 Therefore, Required y = -9 or 3 Class 10 Maths Ex 7.1 Question 9If Q(0, 1) is equidistant from P(5, -3) and R(x, 6), Find the value of x. Also find the distance QR and PR. Solution: Given that, Distance QR = Distance QP ⇒ \sqrt{(x - 0)² + (6 - 1 )²} = \sqrt{(5 - 0)² + (-3 - 1)²} ⇒ x² + 25 = 25 + 16 ⇒ x² = 16 So, x = ±√16 = ±4 Now, For x = 4 QR = \sqrt{(4 - 0)² + (6 - 1 )²} = \sqrt{16 + 25} = \sqrt{41} PR = \sqrt{(x - 5)² + (6 - (-3) )²} = \sqrt{(4 - 5)² + (6 +3 )²} = \sqrt{1 + 81} = \sqrt{82} For x = -4, QR = \sqrt{(-4 - 0)² + (6 - 1 )²} = \sqrt{16 + 25} = \sqrt{41} PR = \sqrt{(x - 5)² + (6 - (-3) )²} = \sqrt{(-4 - 5)² + (6 +3 )²} = \sqrt{81 + 81} = 9 \sqrt{2} Therefore, Required value of x = ±4 and QR = √41 and PR = √82 or 9√2 Class 10 Maths Ex 7.1 Question 10Find a relation between x and y such that the point (x, y) is equidistant from the points (3, 6) and (-3, 4). Solution: Given that, Distance between (3, 6), (x, y) = Distance between (-3, 4), (x, y) ⇒ \sqrt{(x - 3)² + (y - 6)²} = \sqrt{(x - (-3))² + (y - 4)²} ⇒ (x – 3)² + (y – 6)² = (x +3)² + (y – 4)² [squaring both sides] ⇒ x² – 6x + 9 + y² -12y + 36 = x² + 6x + 9 + y² – 8y + 16 ⇒ – 6x – 6x -12y + 8y + 45 – 25 = 0 ⇒ – 12x – 4y + 20 = 0 [divide both side by -4] ⇒ 3x + y – 5 = 0 — Which is the required condition. |
Distance Formula:
The particular formula to find the distance between any two co-ordinate points is known as the “distance formula”. Let us imagine two co-ordinate points as A(x1, y1) and B(x2, y2), Then the distance between the two pints A and B is given by the following formula–
| AB = \sqrt{(x2 - x1)² + (y2 - y1)²} |
–This formula is known as the distance formula.
Proof of Distance Formula:
Let us consider two points A(x1, y1) and B(x2, y2) in co-ordinate plane. We draw perpendicular on X-axis from A and B as AP and BQ. Also draw AM ⊥ BQ.
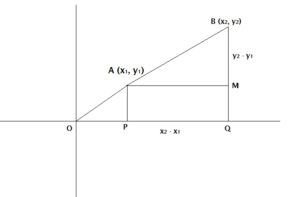
Here, AM = PQ = OQ – OP = x2 – x1
Also, BM = BQ – MQ = y2 – y1
Now, In ΔABM, ∠M = 90°
So, using Pythagoras Theorem on ΔABM,
AB = Distance between the two points
= \sqrt{AM² + BM²}
= \sqrt{(x - 5)² + (6 - (-3) )²}
= \sqrt{(x2 - x1)² + (y2 - y1)²}
Hence the proof.
Special Case of Distance Formula:
If we need to find the distance between any one points from the origin (0, 0), then we can deduce a shortcut formula from the distance formula. Let A(x, y) be any points. Then the distance between A and origin O will be-
| AO = \sqrt{x² + y²} |
Co-ordinate Geometry General Knowledge:
Some most important facts of Co-Ordinate Geometry are given bellow-
- Co-ordinate of origin is (0, 0)
- Co-ordinate of a point on X-axis is (x, 0)
- Co-ordinate of a point on Y-axis is (0, y)
- Distant from X-axis to (x, y) point is = y unites
- Distant from Y-axis to (x, y) point is = x unites
- Co-ordinate of a point in 1st Quadrant = (x, y)
- Co-ordinate of a point in 2st Quadrant = (-x, y)
- Co-ordinate of a point in 3st Quadrant = (-x, -y)
- Co-ordinate of a point in 4st Quadrant = (x, -y)
- Equation of a X- axis is: y = 0
- Equation of a Y- axis is: x = 0
- Equation of a line parallel to X-axis: y = a
- Equation of a line parallel to Y-axis: x = b
ADDITIONAL QUESTION from Class 10 Maths Exercise 7.1 solutions:
In addition to the Class 10 Maths Exercise 7.1 solutions , we have included some most important questions for your final exams bellow. Try to solve them yourself.
- Find the relation between x and y if the point (x, y) is equidistant from (7, 1) and (3, 5)
- Find the point on Y-axis, if it is equidistant from (6, 5) and (-4, 3)
NCERT SOLUTIONS FROM CLASS 10 MATHS :
We are providing complete Class 10 Maths Solution . You can get complete and updated solution from our website. Please click on your prefered chapter bellow from NCERT CLASS 10 MATHS and see the solutions. You can get complete ncert solutions for class 10 maths from here.
| 1 Chapter 1 Real Numbers |
| 2 Chapter 2 Polynomials |
| 3 Chapter 3 Pair of Linear Equations in Two Variables |
| 4 Chapter 4 Quadratic Equations |
| 5 Chapter 5 Arithmetic Progressions |
| 6 Chapter 6 Triangles |
| 7 Chapter 7 Co-ordinate Geometry |
| 8 Chapter 8 Introduction to trigonometry |
| 9 Chapter 9 Application of Trigonometry |
| 10 Chapter 10 Circle |
| 11 Chapter 11 Constructions |
| 12 Chapter 12 Area Related to Circles |
| 13 Chapter 13 Surface area and volumes |
| 14 Chapter 14 Statistics |
| 15 Chapter 15 Probability |
Additional Links:
- THAN vs THEN – Difference Between Than and Then with Examples.
- SSC MTS 2019 PAPER 2 Results date and links @ssc.nic.in
- WHAT IS TRIGONOMETRY? (Trigonometry Complete Course – Click Here)
- Assam HSLC 2020 Results date, all working links to check results online.
- SEBA HSLC Question Paper Collection.
- Irrigation ASSAM 2020 Result, Final Merit List PDF Download!
- HS ASSAM 2020 Science, Arts and Commerce Final Results Online Check Now!
- DEE ASSAM LP UP 1st Provisional Merit List PDF Download HERE!
- CTET OFFICIAL WEBSITE CLICK HERE
Class 10 Maths Exercise 7.1 solutions
Dear sir
My name is Promod
Sir , please upload chapter 7. Ex- 7.2
In your website
Thank you for your help SIR
Hi, we have updated the complete solutions from class 10 ex 7.2 in our website. Please access the page from homepage. Thanks! Please share.